
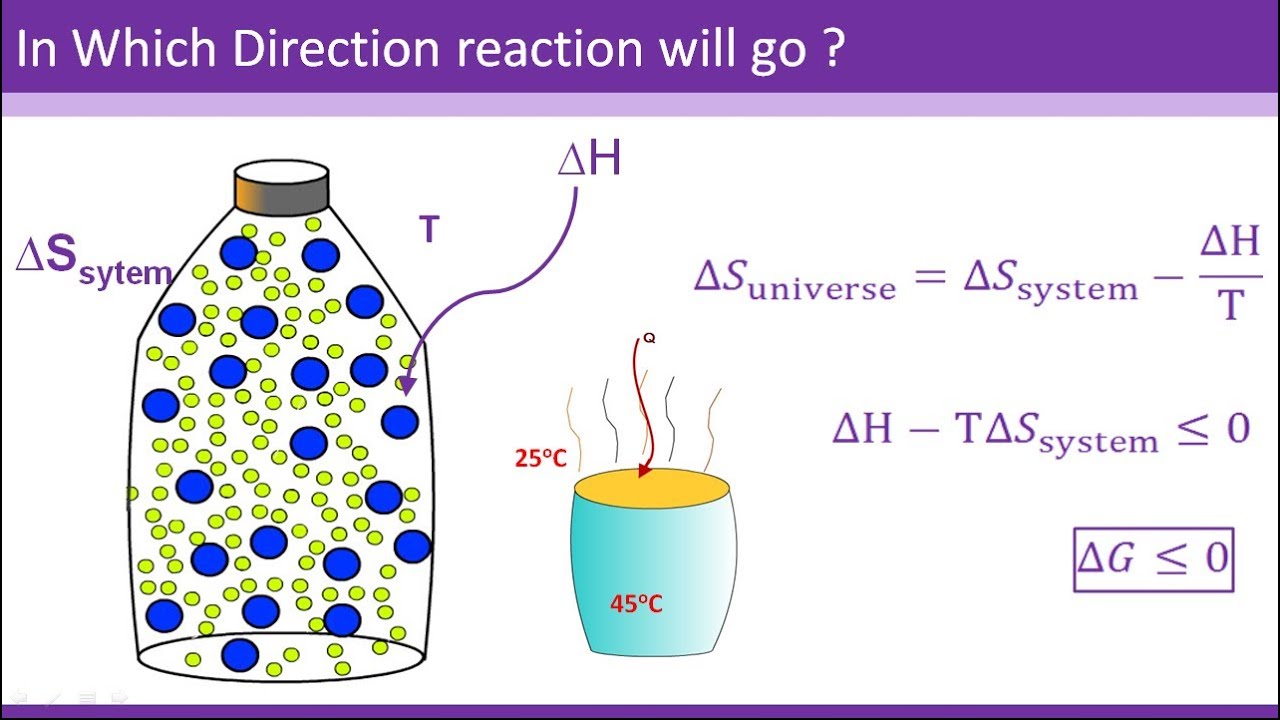
(He’s so modest, he calls the equality the nonequilibrium fluctuation relation, while the rest of us call it Jarzynski’s equality.) Although the DNA experiment provided one of the most famous tests of this principle, the equation governs loads of systems, including those involving electrons, beads the size of bacteria and brass oscillators that resemble centimeter-long tire swings.įluctuation relations have implications fundamental and practical. My colleague Chris Jarzynski at the University of Maryland discovered this in 1997. This is the core of fluctuation relations: Properties of a system far from equilibrium participate in an equality with equilibrium properties. And that relation can be captured by an equality. It turns out that these probabilities - which describe the DNA when it’s far from equilibrium - are directly related to properties that the DNA has at equilibrium. That means every possible amount of work has some probability of being needed during the next pull. The amount of work required fluctuates from one pulling of the strand to the next, since a water molecule sometimes kicks the strand here, sometimes there. Stretching the strand jolts it out of equilibrium and requires work in the physics sense of the word: structured energy harnessed to accomplish a useful task. Using lasers, we can hold one end of the strand steady and pull the other end. Floating quietly, the DNA is at equilibrium, sharing the water’s temperature. To see fluctuation relations in action, imagine a microscopic strand of DNA floating in water. They connect properties of systems far from equilibrium (which are difficult to reason about theoretically) with equilibrium properties (which are easy to reason about). These equalities are known as fluctuation relations. For decades, physicists have worked with equalities that strengthen the second law. Imposing laws on the wild west - meaning, for us, proving equalities about physics far from equilibrium - is quite difficult.īut it’s not impossible. And “far from equilibrium” is the wild west to theoretical physicists and chemists: unpredictable and untidy. This simple, general equality provides precise information about many different types of thermodynamic systems at equilibrium.īut you and I and most of the world are far from equilibrium. (For example, our cake’s scent molecules reach equilibrium after they’ve fully filled the kitchen.) At equilibrium, the second law strengthens to an equality: $latex S_f = S_i$. That happens when large-scale properties - such as temperature and volume - remain constant, and no net flows of anything - such as energy or particles - enter or leave the system. The inequality is useful but vague, because it doesn’t tell us how much the entropy will grow, except in a special case: when the molecules are at equilibrium. We sum up this behavior in an inequality: $latex S_f \ge\ S_i$, where $latex S_i$ is the molecules’ initial entropy and $latex S_f$ their final entropy. Accordingly, the scent of sponge cake wafts across the entire kitchen and never recedes.
ENTROPY LAW OF THERMODYNAMICS WINDOWS
The second law of thermodynamics decrees that the entropy of every closed, isolated system (such as our kitchen, assuming the windows and doors are shut) grows or remains constant. If the volume encompasses the whole kitchen (after the molecules have had time to travel farther), the entropy is exponentially larger. If the volume just encloses the cake (as it does when the cake is freshest), the entropy is relatively small. A physicist might wonder: In how many ways can these molecules be arranged throughout the volume of space they currently occupy? We call this number of arrangements the molecules’ entropy. Scent molecules carrying heat drift away from the cake. To understand its second law, imagine a sponge cake, fresh from the oven, cooling on a countertop. Thermodynamics - the study of energy - originated during the 1800s, as steam engines drove the Industrial Revolution. Since the steam engine began modernizing the world, the second law of thermodynamics has reigned over physics, chemistry, engineering and biology.


 0 kommentar(er)
0 kommentar(er)
